home
contents Mathematics		 next : 
the measurement of the deformation of a contrast
direct to all other pages of the section ----> 00 01 02 03 10 11 12 20 21 30 31 32 33 34

 
 

What is a dimension?



 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
             The key notion developed here, is that numbers not always have one unique dimension: a number such as '2' can as well be the measurement of a length, than the measurement of a surface or the measurement of a volume.
             This does not only mean that you can put a 'm', 'm2' or 'm3' unit behind it, but mainly that according to the number of dimension it bears it must not be calculated in the same way: 2 x 2 will not give the same result if the related '2' have 1, 2, 3 or 4 dimensions.

Before developing this idea, we make a short historic of the notion of dimension.
 
 

From the length of things to the degrees of freedom

In the old time, a dimension was either the height, or the length, or the depth of a thing.

 
In the XVII century, Descartes gave a more general definition : a dimension is a coordinate in a set of orthogonal axes. Height, length and distance, became just special cases of coordinates. Usually these coordinates are said to be that of Euclidean space.
That definition proved to be specially convenient to describe the mechanical movement of a point in space.

In the mid XIX, the mathematician Riemann still gave more generality to the notion of dimension. The physical phenomena evolving by the prompting of various parameters, since Riemann, every parameter that prompts in a process has been call a 'degree of freedom', and every coordinate stands for one of these degrees of freedom. The key point is that nothing prevents us from giving to each point more than three space coordinates, however geometry becomes more complicated each time the number of space dimensions increases.
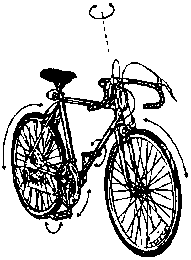
 Here is the example given by Ian Stewart of the dimensions of a motion of a bicycle in his book 'Does God Play Dice?':
"A bicycle has (at a conservative estimate) five main moving parts: the handlebars, the front wheels, the crank-chain-rear-weel assembly, and two pedals. Each requires one position coordinate and one velocity coordinate to describe it: an engineer would say it has 'ten degrees of freedom'. To ride a bicycle, you must gain intuition about the motion of a point in 10-space! Maybe that's what makes it so hard to learn."
[Ian Stewart - Does God Play Dice? - Penguin Books 1990]

 
Therefore, a dimension was at first a concrete part of the things, then a dimension became a coordinate of a moving point in a 3 D space, then a coordinate became a degree of freedom in a 'n' dimensions space, 'n' being as big as needed.

 
 
 

Deterministic chaos and fractal dimensions

These last years, that definition of the dimensions as coordinates of a point was not questioned, but was seriously hit by two events.
For one part, Mandelbrot revealed how useful it was to conceive not whole, but fractional dimensions. A fractional dimension (you must say 'fractal dimension'), is for example 1.3897. To think of a point in a 6 or 10 dimensions space, you can conceive it as an enlargement of a point in a 3 dimensions space: it's more complicated, but it's about the same thing. But what does that mean a coordinate in a 1.3897 dimension space? How can we imagine it? This cannot only be 'more complicated' than a 1 or 2 dimension space, we feel it may be something completely different. At least, it's bizarre.

For the other part, the scientists had to acknowledge that they left aside numerous natural phenomena they cannot calculate. For example, something as simple and ordinary as this one: you throw away a cork in a river and you ask where it will be in an hour. Still in the same place, trapped in a whirlpool? Very far away downstream? May be upstream, drift by a counter-current? May be on the other bank? No equation will help you.
Equations can calculate with breathtaking precision the evolution of a spacecraft lunch in space through the solar system, but they leave you if you want to predict even approximately the evolution of a falling leaf, right in front of your nose, jolted in a very smooth draft.

In the standard scientific jargon, these phenomena insoluble with equations are called: 'deterministic chaos'. Deterministic, for real effects that we can precisely spot and measure determines the future of the events. Chaos, for we know nothing about what's going on despite our knowledge of all what determine the events.
And deterministic chaos appears to be common thing in nature. For instance, water flow and air stream are chaotic in many situations. To have deterministic chaos, you only have to take 3 body and to find there reciprocal influences. The earth round the sun, it's perfectly depicted by an equation, but the earth with the moon and the sun and their respective attractions, already it's fundamentally unpredictable. We can grasp it by tinker equations with approximations and corrections which are not very mathematically limpid, but planets do not go round quickly, and we don't ask what will be in a billion years. To the question: 'is the solar system stable?', no one can tell you with certainty.

The usual explanation is to state that the unpredictability of such phenomenon results of an 'extreme sensibility to initial conditions'. You throw the cork in the river one part of a millimeter left or right, and his travel will be completely different, only by the fact that a very small gap when starting will be magnified during the travel. As time passes, this gap will increase and increase, and finally the cork will be brought in a stationary whirl or in a well-formed current which will rapidly carry it downstream.
It happens that to calculate the motion we have to round off the result of a calculation before beginning the next for it never falls 'right'. Whatever the precision, when we round off it always remains in the result a very tiny gap with the 'right number'. This small gap grows with each of the calculations, and ends being big enough to completely distort the evolution result.
 
 

Toward a new definition of dimensions

 
We shall attempt here to propose a new way of tackle this sort of problem, and to suggest that the powerlessness to describe with an equation the evolution of a point's coordinates in a chaotic phenomenon, doesn't stand in real paradoxes or real unpredictability of phenomena, but simply stands in the inadequacy of our way to represent them, that is to dimension them.
The point is not that we have a problem without solution, it is that we do not put it correctly, and we do not put it correctly because we put it in terms of coordinate dimensions, that is in terms of paths of points whose coordinates evolve in space.

What we will try to show, is that a brand new definition of dimension is needed, more general still than coordinates in a 'n' dimensions space.
In that definition, a coordinate will be only a special sort of dimension, and the path of points will be only a way among others to describe the evolution of a process. Sometimes useful, sometimes inappropriate.
With this definition, fractal dimensions of Mandelbrot will lose their strangeness, and will appear on the contrary more natural and more normal than whole dimensions used to describe a Euclidean space.
 

The usual concept is to describe a dimension as the value we give to a coordinate.
Then, how shall we describe here a dimension?  We state, that a dimension, is the value we give to a deformation.
By and large, we will speak here about 'deformation dimensions'.
What is a deformation dimension?
To first define what the essential link between dimension and deformation is, we shall now demonstrate that some deformations can be measured without ever using any coordinate.

 home 

Math

 top 

 next :  the measurement of the deformation of a contrast

author