home
contents Mathematics
Mandelbrot fractal dimensions to the rescue
continuity of deformations in space
home |
contents Mathematics |
previous
: Mandelbrot fractal dimensions to the rescue |
next
: continuity of deformations in space |
| direct to all other pages of the section ----> | 00 | 01 | 02 | 03 | 10 | 11 | 12 | 20 | 21 | 30 | 31 | 32 | 33 | 34 |
The difference
between fractal and space dimensions
| Now we shall see the crucial point,
where we have to think of the notion of fractal dimensions in the newest
way.
In a space of coordinates, the dimension
1 corresponds to curves, 2 corresponds to surfaces, and 3 corresponds to
volumes.
|
| To think in this way, is to think with old reflexes. |
| A fractal dimension holds 2 parts:
a whole number, then, after a dot, a decimal number.
We have already suggested the signification of these two parts. We now see this question again, in a more precise and a more complete way, beginning with the whole number. |
"0"
: We gave an example [to see ![]() this example again] of a contrast value, measured with spots
on a white sheet, then, in a 2D space. Instead of 2D spots, we could have
taken 3D black bubbles in a 3D white volume: the contrast ratio measuring
the ratio between the volume of the bubbles and the whole space, would
always have been less than 1.
this example again] of a contrast value, measured with spots
on a white sheet, then, in a 2D space. Instead of 2D spots, we could have
taken 3D black bubbles in a 3D white volume: the contrast ratio measuring
the ratio between the volume of the bubbles and the whole space, would
always have been less than 1.
Therefore, a fractal value smaller
than 1, whose 1st digit is consequently 0, has nothing to do with the incapacity
to completely fill a line 1D.
Digit 0 only
indicates that the phenomenon implies no displacement in space.
The deformation of the whiteness of a line, of a page, or of a volume,
implies for itself the motion of no single point at all, but it implies
the appearance or the disappearance of an infinite number of points.
Then,
the dimensions with 0 as whole value are specially convenient for the measurement
of mutations:
what is uncolored becomes colored, what is young becomes old, what is inert
becomes lively, what is healthy becomes sick, etc.
But we cannot see a move explaining
the turning of a young person into an old one: before becoming old, and
old person is nowhere among the old persons. Then one day he or she appears
with this property, coming from nowhere, and one day he or she dies, disappearing
with this property from the old persons.
"1"
: When we gave some examples of fractal curves (for instance ![]() Van Kock's snowflake curve), we saw that when the deformation implies a
displacement in space, then the whole value of the fractal dimension is
1.
Van Kock's snowflake curve), we saw that when the deformation implies a
displacement in space, then the whole value of the fractal dimension is
1.
This
type of dimension is uniquely convenient to describe the moving of a body.
It is not convenient to describe
or to predict the possible changes of its property while moving, which
fits to the dimension 0 we just saw.
Nor is it convenient to describe
its possible deformations while moving, which fits to the dimension 2 we
see now.
"2"
: We have already suggested that the whole value 2 of the Peano curve
[see
below its representation] has to do with the deformation of a surface
on itself. More generally we suggest now that the whole value 2 in a dimension
has to do with the deformation of a body on itself. With
this term we mean the coordinated internal displacement of all its points,
all exchanging one another, without the global displacement of the body
in space.
In the section "science"
of the french site, we suggest that a particle of matter is the result
of a very complex movement that works in a close loop, which is exactly
a case of internal deformation. So,
all what is related to the stability of matter particles and their internal
coherence is specially concerned by this type of dimension.
Laurent Nottale who tried to combine
quantum mechanics of elementary particles with fractal calculation, end
up with the same conclusion.
We translate an extract of one of
its article ['L'espace-temps fractal' - Pour la Science - September
1995];
'The two dimension is, precisely,
those of the fractal trajectory calculated from Heisenberg's uncertainty
relations'
| To sum up, our hypothesis is
that if the fractal dimension is 0,x then it's a contrast value, if the
fractal dimension is 1,x then it's the value of a trajectory, and if the
fractal dimension is 2,x then it's the value of an internal deformation.
But here too, like what we saw for
the value 0, we emphasize that the values 1 or 2 have nothing to do with
the number of the dimensions of the space where the phenomenon occurs.
|
Then, if the whole number gives no
information about the evolution of the deformation in space, decimal digits
have to do.
In that respect we make the hypothesis that
the decimal value of a fractal dimension indicates how the intensity of
the deformation varies according to space directions.
| If the deformation is restricted to a line or to a surface, the decimal series will do in such a way that the deformation value will be null in all the forbidden directions. |
Another significant point, which results from the incommensurability between whole part, and decimal part of a fractal dimension: the whole part has nothing to do with the intensity of the deformation.
| For example, a 2.1 dimension is
in any way a more intense deformation than a 1.1 dimension.
This is fundamental for the comparison of fractal dimensions the ones with the others: for example, a dimension 1.999 . . . . and so on, is not a dimension very close to dimension 2. Rather, they are bound to be very far away from each other: one is an extremely tortuous path that can occur even in a 3D space, whereas the other can concern the deformation of a surface only, even of a curve only. [see the example below] |
But here is a new important subtlety:
we can think of a dimension with a value 2, in two completely distinct
ways.
Either we say that it's a dimension
2.0, that is, for example, the regular deformation of a surface on itself,
such as made with the evolution of a 'Peano curve'.
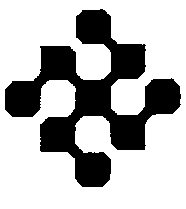 |
==> |  |
==> |  |
etc |
Either we say that it's the limit
at infinity to a dimension 1.99999 (with 9 till infinity). That is, a path
which is subjected to the maximum roundabouts that a path can be subjected
to, roundabouts that are systematically and completely random.
This has nothing to do with a surface.
This, is precisely the fractal value of what is called the 'Brownian movement',
the movement of gas molecules, moving absolutely randomly in all space
directions. Then, with maximum irregularity in their path. Doing this,
they move within a 3 D volume, not on a 2 D surface.
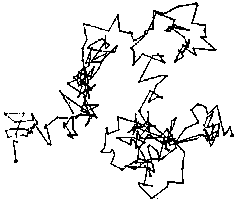 a
sample of Brownian movement: a
sample of Brownian movement:
its fractal dimension is 2 but its signification would be 1.9999999 till infinity |
We can also consider mixing space dimensions
and fractal dimensions.
Thus, a Menger sponge [to
see ![]() this figure again], has a fractal value Log 20 / Log 3, close
to 2.7268.
this figure again], has a fractal value Log 20 / Log 3, close
to 2.7268.
The whole part '2' would not correspond
in this case with the information to the type of the deformation. Its type
corresponds to the dimension 0, for the generation of this volume is similar
to that of a Cantor dust.
The value 2.7268 corresponds to
the evolution of the surface separating empty parts and full parts of the
volume. It has to do with the conversion in terms of surface (then the
value 2D) of a process of generation with dimension 0,x.
| In the end, if fractal dimensions
are more general than space dimensions, it implies that space dimensions
are only fractal dimensions with special values.
Let's calculate their value: On the axes of measurement of the Euclidean space, the coordinates follow straight paths. Being paths, they deserve 1 as whole fractal value. As these paths do remain straight ones and are subjected to none deformation in any direction, they deserve the decimal value 0. In short, fractal dimensions are not special and partial space dimensions, on the opposite space dimensions are only a special and limited case of fractal dimensions, with the very special value 1.0. |
home |
Math |
top |
next
: |
author |