home
contents Mathematics
to see what's going on in the 4th dimension
home |
contents Mathematics |
previous
: to see what's going on in the 4th dimension |
| direct to all other pages of the section ----> | 00 | 01 | 02 | 03 | 10 | 11 | 12 | 20 | 21 | 30 | 31 | 32 | 33 | 34 |
Chains of dimensions
When a line is being drawn, as the
time passes by its points jump from one position to another position of
this line. In a strange attractor,
points do not jump anymore on a line but jump on a surface.
So, we
only have to acknowledge the evidence: a strange attractor does not
represent the evolution of a line, but that of a surface.
We shall make the hypothesis, that
this mutation of the diagram along with the increase of the number of its
dimensions (a 1D line being replaced by a 2D surface) would have link with
the mutation of the represented fractal dimension: a line would represent
a fractal dimension '1', and a surface would represent a fractal dimension
'2'.
But we cannot understand the meaning
of this mutation if we only consider the functioning of a strange attractor,
and we now have to suggest a general hypothesis on the chain of all types
of dimensions. To present our hypothesis, we sum it up in a tabular form.
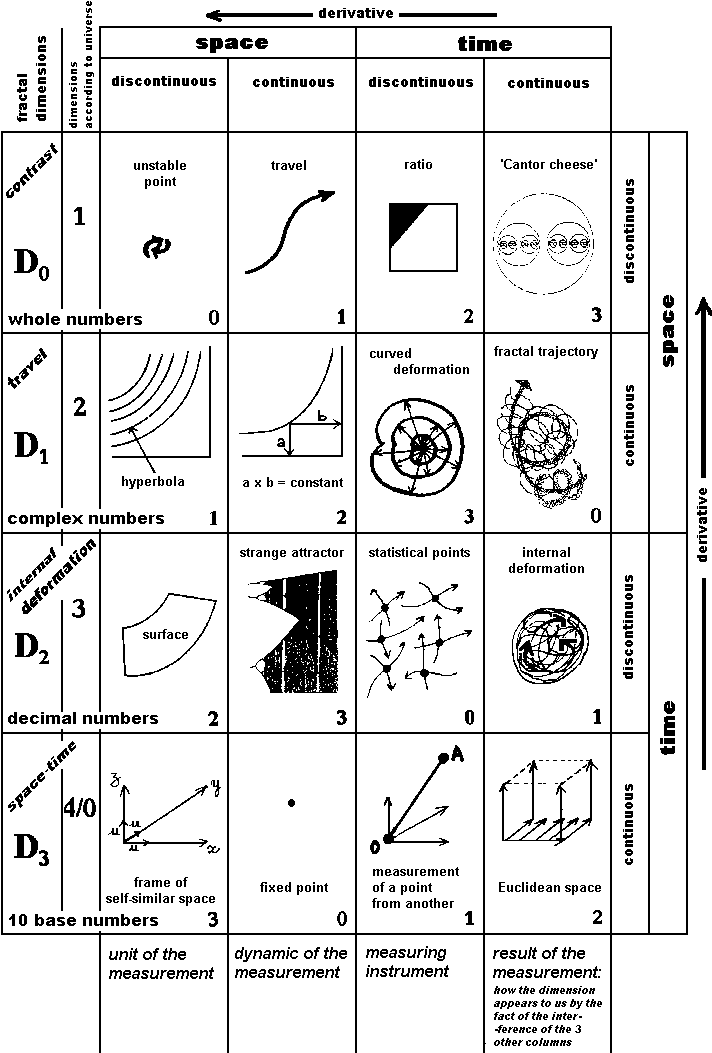
Every horizontal line corresponds to fractal dimension, from 0 to 3.
-
in the 1st line, we
find the fractal dimension whose whole part is 0.
We said it's specially convenient for the measurement of a contrast [to
see this ![]() again].
again].
The first two squares of this line
show a functioning which is similar to what we described for the generating
process of whole numbers: the instability of 0, whose vibration unwind
in one go all whole numbers till infinity [to
see again ![]() the chapter "Lets' go back from zero"].
the chapter "Lets' go back from zero"].
Then, we will say this '0' fractal
dimension is that of whole numbers.
-
in the 2nd line, we
find the fractal dimension whose whole part is 1.
We said it's specially convenient for the measurement of the motion of
a body [to see this ![]() again].
again].
This dimension works by the simultaneous
measurement of two values. As this characteristic is also that of complex
numbers, we will say this '1' fractal dimension is that of complex numbers.
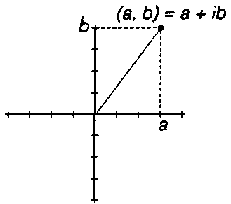 |
complex
numbers can be written down
in the form a + ib (where i is the square root of -1) or be represented by a couple of numbers |
-
in the 3rd line, we
find the fractal dimension whose whole part is 2.
We said it's specially convenient for the measurement of the deformation
of a body on itself [to see this ![]() again].
again].
In the chapter "Let's go back from
zero" [to see this chapter ![]() again],
we explained why decimal numbers need a '2' dimension to be produced and
recorded. Then we will say this '2' fractal dimension is that of decimal
numbers.
again],
we explained why decimal numbers need a '2' dimension to be produced and
recorded. Then we will say this '2' fractal dimension is that of decimal
numbers.
-
in the 4th line, we
find the fractal dimension whose whole part is 3.
Until now we did not suggest a meaning for this type of dimension.
Now, we suggest that this dimension
is that of usual coordinates in a space-time diagram.
The '3' dimension being specialized
in the interference between previous dimensions, we propose to consider
it to be the dimension that enables the combining of whole numbers with
complex numbers and with decimal numbers.
As the 10 base numbers make possible
to easily calculate numbers in all situations, we will say this '3' fractal
dimension is that of 10 base numbers.
| Then the 1st line
corresponds to fractal dimensions with 0 for whole part.
As we suggest, these dimensions are to calculate contrasts. |
| In every corner of every square
of the table, we put a dimension value which enables to read the table
in a diagonal order. This reading is thus crossed with the progress along
the lines and with the progress along the columns.
We note that on every diagonal, from the left bottom to the right top, the corner dimensions are identical. This means that diagonal progress is well balanced for the reading of all squares when we go from the left top to the right bottom. When we find the digit '3',
this means that we are in the square where the self-similar character of
the fractal dimension is working.
When we find the digit '2',
this means that we are in the square where the decimal value of the fractal
dimension is working.
When we find the digit '1',
this means that we are in the square where the complex function of the
fractal dimension is working.
When we find the digit '0',
this means that we are in the square where the whole value of the fractal
dimension is working.
|
| The 2nd line
corresponds to fractal dimensions with 1 for whole part.
These dimensions are specially convenient to calculate the movements. |
| The 3rd line
corresponds to fractal dimensions with 2 for whole part.
These dimensions are specially convenient for the measurement of internal deformations. |
| We can understand as follows
that the unit of measurement of this dimension is a surface:
We have to measure the deformation of a body on itself, and this needs that all the points of the body permanently coordinate together to exchange their respective places. In effect, during all the deformation two points must never occupy the same place, for this would let empty places, holes in the deserted areas, and would make excessive density in the over-occupied areas. Then, every point follows its one way, what corresponds to a '1' dimension, and is involved in the same time in the density dimension of the body so that this density remains constant. It happens that a density dimension is a contrast dimension, then a '0' dimension. Every point is then subjected
to the simultaneous effect of 2 dimensions of a different type: one is
a '1' dimension, and the other is a '0' dimension.
Two dimensions, then it makes a surface.
|
| The 4th and last
line corresponds to fractal dimensions with 3 for whole part.
These dimensions are the usual dimensions of space-time. |
At the end of all these reflections,
the traditional concept of measurement of space with 3 graduated orthogonal
axis, appears to be a method within four radically different methods to
measure the phenomena: four methods that are complementary the ones for
the others, and that are all contained the ones in the others.
What we finally discover is that
a
dimension is nothing but one of the 4 ways we have to combine 4 dimensions,
nothing but one of the 4 ways we have to permute their complementary roles.
The interest of this table could be to help find the possible 'nonprobability' measurement of the fractal dimension '2'.
Generally, the idea would be to think how every dimension is the 'derivative'
of the just above dimension, and the 'primitive' of the just below dimension.
The handling of the derivatives
by Newton and Leibniz was the mathematic tool that made possible all the
development of the scientific calculation since the XVII century.
Today, we still consider that a
derivative is the instantaneous change of the direction of a curve: it
would be the limit of this change when time duration tends to zero. This
concept is effective, but it is not convenient to think that a change can
really be made in zero time: in zero time a change can only be zero.
As our hypothesis proposes that
dimensions are fundamentally only deformations, it does not have this abnormality:
we consider a travel as the coordination of 2 deformations, and we can
very well stop one of the deformation making it zero, and then measuring
the other deformation that has not to be specially zero at the same time.
In
our hypothesis we call 'derivative' of a curve, the value of one of the
deformations of a travel, when its combined dimension is zero.
The dynamic of the '2' deformation
has a 'volumetric' aspect that provokes its statistical feature, for we
cannot calculate the edge of a parallelepiped if we only know its volume.
I hope
the analysis of this table will enables somebody
to find the evolution
of the surface of one of the sides of this parallelepiped, finding by this
way the absolute length of its edges.
Last update of this page: 09 mai 2010
home |
Math |
top |
author |