
 of this text]
of this text]| The First Paradoxical Effect: "homogeneous/heterogeneous" |
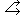 ".
Of course, there is no use to remember this numbering. You just have to
notice that "a" means "analytic". "3" is the numbering of another
paradox which is connected to this effect and which doesn't matter for
the moment.
".
Of course, there is no use to remember this numbering. You just have to
notice that "a" means "analytic". "3" is the numbering of another
paradox which is connected to this effect and which doesn't matter for
the moment. 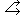 is the graphic symbol for the paradox homogeneous/heterogeneous. A page indicates how the different symbols were chosen.
is the graphic symbol for the paradox homogeneous/heterogeneous. A page indicates how the different symbols were chosen. link, you will have other examples of works in which some part is
homogeneously made and the other one is heterogeneously made. Although
on the same principle, you will see that the means are different: as
here, sometimes it would be homogeneous surfaces contrasting with
the heterogeneity of the intersections between these surfaces, but
sometimes it would be the homogeneity of a simple line in its usual
part contrasting with the sharp folds of its local inflections, or
it would be the homogeneity of a texture of lines contrasting with
completely irregular, then heterogeneous textures.
link, you will have other examples of works in which some part is
homogeneously made and the other one is heterogeneously made. Although
on the same principle, you will see that the means are different: as
here, sometimes it would be homogeneous surfaces contrasting with
the heterogeneity of the intersections between these surfaces, but
sometimes it would be the homogeneity of a simple line in its usual
part contrasting with the sharp folds of its local inflections, or
it would be the homogeneity of a texture of lines contrasting with
completely irregular, then heterogeneous textures. .
Clicking on the link of this numbering, you can then discover its use
in other works of art. Of course, "s" is a reminder for "synthetic".
.
Clicking on the link of this numbering, you can then discover its use
in other works of art. Of course, "s" is a reminder for "synthetic".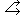 and the s10
and the s10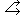 links reveal the return of this effect in other periods of art history? And why not in the whole periods?
links reveal the return of this effect in other periods of art history? And why not in the whole periods?| Second Paradoxical Effect. We will call it: "to assemble/to separate" |
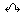 ) which will lead you to other examples of works using similar effects.
) which will lead you to other examples of works using similar effects.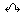 type of expression we do not find this relation
in the global arrangement of the shapes, but we find it in the internal
relation of the different shapes: in a place the shapes gather
together, whereas they move away from each other in another place.
type of expression we do not find this relation
in the global arrangement of the shapes, but we find it in the internal
relation of the different shapes: in a place the shapes gather
together, whereas they move away from each other in another place. We can also find this effect in the breasts, assembling at the top in the chest, and separating toward the bottom.
We can also find this effect in the breasts, assembling at the top in the chest, and separating toward the bottom.| Third Paradoxical Effect. We will call it: "synchronized/incommensurable" |
| Fourth Paradoxical Effect. We will call it: "continuous/cut" |
|
|
State Paradoxes And Transformation Paradoxes |
| The First Transformation Paradox: "coordinated effect/autonomy" |
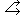 expression of the paradox homogeneous/heterogeneous (second transformation paradox) used in the Venus.
expression of the paradox homogeneous/heterogeneous (second transformation paradox) used in the Venus.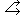 expression of the paradox homogeneous/heterogeneous used in the Venus.
expression of the paradox homogeneous/heterogeneous used in the Venus.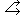 expression of the paradox homogeneous/heterogeneous, in the first and in the second example we gave of its use in the Venus.
expression of the paradox homogeneous/heterogeneous, in the first and in the second example we gave of its use in the Venus.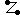 expression also uses the lozenge shape:
expression also uses the lozenge shape: expression of the paradox homogeneous/heterogeneous.
expression of the paradox homogeneous/heterogeneous.|
|
Before Leaving The Venus Of Lespugue |
home |
top |
next: |
|